When A Is Changing At A Rate Of -0.160 Mã¢â€¹â€¦sã¢ë†â€™1 , How Fast Is B Changing?

2.5: Reaction Rate

1. Last updated

2. Relieve as PDF

Page ID

1438

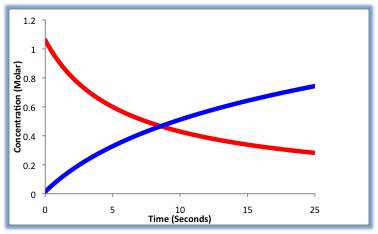
During the course of the reaction shown below, reactants A and B are consumed while the concentration of product AB increases. The reaction charge per unit can be adamant past measuring how fast the concentration of A or B decreases, or by how fast the concentration of AB increases.

\[ \ A + B \longrightarrow AB \]

Figure \(\PageIndex{ane}\): The above motion-picture show shows a hypthetical reaction profile in which the reactants (red) decrease in concentration equally the products increase in concetration (bluish).

For the stochiometrically complicated Reaction:

\[ aA + bB \longrightarrow cC + dD \characterization{i} \]

\[ \text{Charge per unit} = \dfrac{-i}{a}\dfrac{d[A]}{dt} = \dfrac{-1}{b} \dfrac{d[B]}{dt} = \dfrac{1}{c}\dfrac{d[C]}{dt} = \dfrac{1}{d}\dfrac{d[D]}{dt} \]

Looking at Effigy \(\PageIndex{1}\) above, we can encounter that the rate can be measured in terms of either reactant (A or B) or either product (C or D). Non all variables are needed to solve for the charge per unit. Therefore, if you take the value for "A" besides as the value for "a" yous can solve for the reaction rate.

You can also notice from Equation \ref{1} that the modify in reactants over the change in time must have a negative sign in front of them. The reason for this is because the reactants are decreasing as a function of time, the rate would come up out to be negative (because information technology is the reverse rate). Therefore, putting a negative sign in front of the variable will permit for the solution to be a positive rate.

Chemical reactions vary greatly in the speed at which they occur. Some are ultrafast, while others may take millions of years to reach equilibrium.

Definition of Reaction Rate

The Reaction Rate for a given chemical reaction is the measure out of the change in concentration of the reactants or the modify in concentration of the products per unit time. The speed of a chemic reaction may be defined as the change in concentration of a substance divided by the time interval during which this modify is observed:

\[ \text{charge per unit}=\dfrac{\Delta \text{concentration}}{\Delta \text{time}} \characterization{2-1}\]

For a reaction of the class \(A + B \rightarrow C\), the rate can exist expressed in terms of the alter in concentration of any of its components

\[\text{charge per unit}=-\dfrac{\Delta [A]}{\Delta t}\]

\[\text{rate}=-\dfrac{\Delta [B]}{\Delta t}\]

\[\text{rate}=\dfrac{\Delta [C]}{\Delta t}\]

in which \(Δ[A]\) is the divergence betwixt the concentration of \(A\) over the fourth dimension interval \(t_2 – t_1\):

\[ \Delta [A] = [A]_2 – [A]_1 \label{2-two}\]

Discover the minus signs in the outset two examples to a higher place. The concentration of a reactant always decreases with time, so \(\Delta [A]\) and \(\Delta [A]\) are both negative. Since negative rates do non make much sense, rates expressed in terms of a reactant concentration are ever preceded by a minus sign to brand the charge per unit come out positive.

Consider now a reaction in which the coefficients are different:

\[A + 3B \rightarrow 2D\]

It is clear that \([B]\) decreases three times every bit rapidly as \([A]\), and so in order to avoid ambivalence when expressing the rate in terms of different components, it is customary to separate each change in concentration by the appropriate coefficient:

\[\text{rate}= -\dfrac{\Delta [A]}{\Delta t} = -\dfrac{\Delta [B]}{3\Delta t} = \dfrac{\Delta [D]}{2\Delta t} \label{ii-3}\]

Example \(\PageIndex{1}\): Oxidation of Ammonia

For the oxidation of ammonia

\[\ce{4 NH3 + 3O2 -> ii N2 + half dozen H2O}\]

it was plant that the rate of formation of N₂ was 0.27 mol L^–ane s^–1.

1. At what charge per unit was water being formed?
2. At what rate was ammonia being consumed?

Solution

a) From the equation stoichiometry, Δ[H_twoO] = 6/two Δ[Northward₂], and so the rate of formation of H₂O is

three × (0.27 mol L^–1 due south^–i) = 0.81 mol L^–1 s^–i.

b) 4 moles of NH₃ are consumed for every ii moles of North₂ formed, then the rate of disappearance of ammonia is

2 × (0.27 mol L^–1 south^–1) = 0.54 mol L^–i southward^–one.

Comment: Because of the way this question is formulated, information technology would exist acceptable to express this last value equally a negative number.

Instantaneous rates

About reactions tedious down every bit the reactants are consumed. Consequently, the rates given by the expressions shown higher up tend to lose their meaning when measured over longer time intervals Δt. Note: Instantaneous rates are also known equally differential rates.

Thus for the reaction whose progress is plotted here, the bodily rate (as measured by the increasing concentration of product) varies continuously, existence greatest at time naught. The instantaneous charge per unit of a reaction is given by the slope of a tangent to the concentration-vs.-time bend.

An instantaneous rate taken most the beginning of the reaction (t = 0) is known as an initial rate (label (one) here). As nosotros shall soon see, initial rates play an of import role in the written report of reaction kinetics. If you take studied differential calculus, yous will know that these tangent slopes are derivatives whose values can very at each point on the curve, then that these instantaneous rates are really limiting rates defined equally

\[ \text{charge per unit} = \lim_{\Delta t \rightarrow 0} \dfrac{-[A]}{\Delta T}\]

If you practise non know calculus, bear in listen that the larger the time interval Δt, the smaller will be the precision of the instantaneous rate.

Rate Laws and Rate Constants

A rate law is an expression which relates that rate of a reaction to the charge per unit constant and the concentrations of the reactants. A rate abiding, \(k\), is a proportionality constant for a given reaction. The general rate police force is usually expressed as:

\[ \text{Charge per unit} = k[A]^due south[B]^t \label{2}\]

As you can see from Equation \ref{2} in a higher place, the reaction charge per unit is dependent on the concentration of the reactants too as the rate constant. However, there are besides other factors that can influence the rate of reaction. These factors include temperature and catalysts. When you are able to write a rate law equation for a sure reaction, you can determine the Reaction Order based on the values of south and t.

Reaction Order

The reaction rate for a given reaction is a crucial tool that enables us to calculate the specific order of a reaction. The society of a reaction is important in that information technology enables us to allocate specific chemical reactions hands and efficiently. Knowledge of the reaction order quickly allows us to understand numerous factors within the reaction including the rate constabulary, units of the rate constant, half life, and much more. Reaction order can be calculated from the rate constabulary by adding the exponential values of the reactants in the rate law.

\[ \text{Rate} = k[A]^s[B]^t \characterization{iv}\]

\[ \text{Reaction Order} = s + t \label{v}\]

It is important to note that although the reaction lodge can exist adamant from the rate law, there is in full general, no relationship between the reaction lodge and the stoichiometric coefficients in the chemical equation.

Note: The rate of reaction must be a not-negative value. It can be cypher and does not need to be an integer.

As shown in Equation \ref{five}, the complete reaction order is equal to the sum of "due south" and "t." But what does each of these variables mean? Each variable represents the order of the reaction with respect to the reactant information technology is placed on. In this certain state of affairs, s is the order of the reaction with respect to [A] and t is the order of the reaction with respect to [B].

Here is an example of how yous tin can wait at this: If a reaction society with respect to [A] was ii (south = two) and [B] was one (t = 1), then that basically means that the concentration of reactant A is decreasing past a gene of 2 and the concentration of [B] is decrease past a cistron of 1.

Then if you have a reaction gild of Zero (i.e., \(due south + t = 0\)), this basically means that the concentration of the reactants does not bear on the charge per unit of reaction. You could remove or add together reactants to the mixture simply the rate will not change.

A listing of the unlike reaction rate equations for cipher-, first-, and second-order reactions tin can be seen in Tabular array \(\PageIndex{1}\). This tabular array also includes further equations that tin be determine past this equation in one case the order of the reaction is known (Half life, integrated rate law, etc.)

Table \(\PageIndex{1}\): The table below displays numerous values and equations utilized when observing chemic kinetics for numerous reactions types

	Cipher-Order	Offset-Lodge	2d-Order
Charge per unit Police	\(\ {Rate} = \ {thousand}\)	\(\ {Rate} = \ {k[A]}\)	\(\text{Charge per unit} = \ {thousand[A]^two}\)
Integrated Rate Police force	\(\ {[A]_t} = \ {-kt + [A]_0}\)	\(\ {ln[A]_t} = \ {-kt + ln[A]_0}\)	\(\dfrac{1}{[A]_t} = -kt + \dfrac{one}{[A]_0} \)
Units of Rate Constant (chiliad):	\(\ {mol L^{-1} southward^{-ane}}\)	\(southward^{-1}\)	\(\ {L mol^{-i} s^{-1}}\)
Linear Plot to Determine (1000):	\( [A] \) versus time	\(\ln [A] \) versus time	\(\dfrac{i}{[A]}\) versus time
Relationship of Rate Constant to the Gradient of Straight Line:	\(\ {slope} = \ {-k}\)	\(\ {slope} = \ {-one thousand}\)	\(\ {gradient} = \ {g}\)
Half-life:	\(\dfrac{[A]_0}{2k}\)	\(\dfrac{\ln2}{k}\)	\(\dfrac{ane}{chiliad[A]_0}\)

Sample Problems

1. Ascertain Reaction Charge per unit

2. TRUE or FALSE: Changes in the temperature or the introduction of a catalyst will affect the rate constant of a reaction

For sample problems iii-6, use Formula 6 to respond the questions

\[ H_2O \longrightarrow 2H_2+ O_2 \label{vi} \]

*Assume the reaction occurs at constant temperature

3. For the given reaction above, state the charge per unit law.

4. Land the overall order of the reaction.

5. Find the rate, given k = 1.14 x 10^-2 and [H_twoO] = two.04M

half-dozen. Detect the one-half-life of the reaction.

Answers

1. Reaction Rate is the mensurate of the alter in concentration of the disappearance of reactants or the change in concentration of the appearance of products per unit of measurement time.

2. FALSE. The rate constant is not dependant on the presence of a catalyst. Catalysts, nevertheless, tin effect the total rate of a reaction.

iii. \(\ {Rate} = \ {yard[H_2O]}\)

4. First - Order

5. 2.33 x 10^-2 s^-1

vi. 60.8 s ((ti/2 = ln 2 / k = ln 2 / ane.14 x 10^-two = 60.eight s).

References

1. Chang, Raymond. (2005). Physical Chemical science for the Biosciences. Sausalito,CA: University Science Books.
2. Crowe, Jonathan, Bradshaw, Tony, MonkPaul. (2006) Chemistry for the Biosciences: The Essential Concepts. Oxford Press.
3. Isaacs, Due north.S. (1995). Physical Organic Chemistry (2nd edition). Harlow United kingdom of great britain and northern ireland: Adison Wesley Longman.
4. Kenneth Connors. (1990). Chemical Kinetics. VCH Publishers.
5. Levine, Ira N. (1988). Physical Chemistry (Third edition). McGraw-Loma Inc.
6. Segel, Irwin. (1993). Enzyme Kinetics. Wiley Classics Library.
7. Zumdahl, Steven S. and Zumdahl, Susan A. (2003) Chemistry (Sixth edition). Houghton Mifflin Co.
8. Shagoury, Richard. Chemistry 1A Lecture Book. 4th Ed. Custom Publishing. 2006. Print

Source: https://chem.libretexts.org/Bookshelves/Physical_and_Theoretical_Chemistry_Textbook_Maps/Supplemental_Modules_(Physical_and_Theoretical_Chemistry)/Kinetics/02%3A_Reaction_Rates/2.05%3A_Reaction_Rate

Posted by: pylantantless.blogspot.com

Share this post